Two-Dimensional Space Groups

Steven Dutch, Natural and Applied Sciences, University of Wisconsin - Green Bay
First-time Visitors: Please visit Site Map and Disclaimer. Use "Back" to return here.

What Kinds of Two-Dimensional Symmetry are Possible?

If we are only concerned about a single point, any symmetry is possible in two dimensions; a flower can have any number of petals. But if the pattern repeats, then the possibilities are very limited. Consider the diagram below:

                  o     o     o     v     q     o     o
                                     \   /
                                     a\ /a
                     o     o     u-----x-----p     o    


                  o     o     o     o     o     o     o

Point x is an axis of n-fold symmetry, and so is every other point. (The pattern repeats, after all.) So point u gets rotated to point v (among others), and point p gets rotated to point q. Now in this repeating pattern, all the points are spaced the same distance apart in the rows. Call that distance 1. Points v and q must be an integer distance apart. In the sketch they are distance 1 apart but in a square lattice, for example, they might coincide. Thus distance vq = an integer = 2 cos a

Now the cosine can only have values between -1 and 1, so 2 cos a, which must be an integer, can only take on values -2, -1, 0, 1 and 2. Thus cos a can only be -1, -1/2, 0, 1/2 and 1, or a = 180, 120, 90, 60 and 0 (or 360), respectively. Since the rotations are symmetry rotations,
a = 360/n, so n = 2, 3, 4, 6, or 1

Thus, repeating patterns in the plane can only have 1, 2, 3, 4 or 6-fold symmetry. In particular, repeating patterns in the plane cannot have five-fold symmetry.

What about the stars in the flag? The stars have five-fold symmetry, but the overall symmetry of the pattern does not.
In recent years some fascinating materials have turned up with five-fold symmetry. These materials, termed quasicrystals, do not have repeating patterns.

What Combinations of Two-Dimensional Symmetry are possible?

In the diagram below, points a, b, c, and d are symmetry axes (not necessarily the same kind in a plane pattern.

                             b
                          y  |  y
                    x        |        x
                 a-----------+-----------d
                    x        |        x
                          y  |  y
                             c

Symmetry axis a rotates b to c and vice versa. Axis b rotates a to d and so does axis c. Finally, axis d rotates b to c and vice versa. The rotated axes have to coincide eventually. If they do not, the entire plane will eventually be covered with an infinite number of symmetry axes. Apart from patterns with only one- and two-fold symmetry, the only possibilities are:

n = 3, m = 3
n = 3, m = 6
n = 4, m = 4

The five basic lattice types

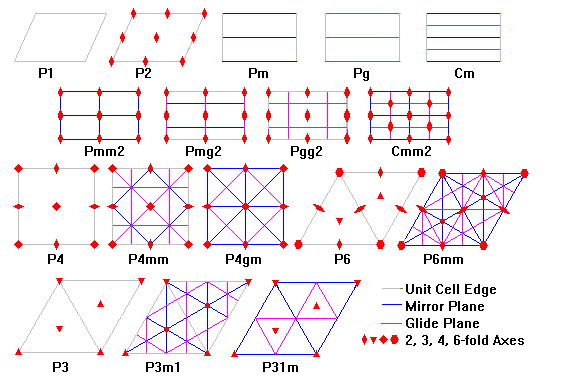
There are 17 space groups in the plane, but their unit cells fall into one of five basic shapes as follows:

General Parallelogram lattice

+-----------+-----------+-----------+-----------+-----------+-----------+
           /           /           /           /           /           /
          /           /           /           /           /           /
         /           /           /           /           /           /
--------+-----------+-----------+-----------+-----------+-----------+---
       /           /           /           /           /           /
      /           /           /           /           /           /
     /           /           /           /           /           /
----+-----------+-----------+-----------+-----------+-----------+-------
   /           /           /           /           /           /
  /           /           /           /           /           /
 /           /           /           /           /           /
+-----------+-----------+-----------+-----------+-----------+-----------+

General Rectangular Lattice

+-----------+-----------+-----------+-----------+-----------+-----------+
|           |           |           |           |           |           |
|           |           |           |           |           |           |
|           |           |           |           |           |           |
+-----------+-----------+-----------+-----------+-----------+-----------+
|           |           |           |           |           |           |
|           |           |           |           |           |           |
|           |           |           |           |           |           |
+-----------+-----------+-----------+-----------+-----------+-----------+
|           |           |           |           |           |           |
|           |           |           |           |           |           |
|           |           |           |           |           |           |
+-----------+-----------+-----------+-----------+-----------+-----------+

General Rhombic (Centered) Lattice

    +       +       +       +       +-------+-------+-------+-------+----
   / \     / \     / \     / \     /|\     /|\     /|\     /|       |
  /   \   /   \   /   \   /   \   / | \   / | \   / | \   / |       |  
 /     \ /     \ /     \ /     \ /  |  \ /  |  \ /  |  \ /  |       | 
+       +       +       +       +   |   +   |   +   |   +   |   +   |   +
 \     / \     / \     / \     / \  |  / \  |  / \  |  / \  |       |
  \   /   \   /   \   /   \   /   \ | /   \ | /   \ | /   \ |       |   
   \ /     \ /     \ /     \ /     \|/     \|/     \|/     \|       |     
    +       +       +       +       +-------+-------+-------+-------+----
   / \     / \     / \     / \     /|\     /|\     /|\     /|       |
  /   \   /   \   /   \   /   \   / | \   / | \   / | \   / |       |  
 /     \ /     \ /     \ /     \ /  |  \ /  |  \ /  |  \ /  |       | 
+       +       +       +       +   |   +   |   +   |   +   |   +   |   +
 \     / \     / \     / \     / \  |  / \  |  / \  |  / \  |       |
  \   /   \   /   \   /   \   /   \ | /   \ | /   \ | /   \ |       |   
   \ /     \ /     \ /     \ /     \|/     \|/     \|/     \|       |     
    +       +       +       +       +-------+-------+-------+-------+----

The simplest unit cell for this pattern is a rhombus, but the pattern also has a rectangular structure. To bring out the rectangular pattern, this lattice is often described as a rectangle with an extra point in the center. Such a lattice is called centered.

Square Lattice

+-------+-------+-------+-------+-------+-------+-------+-------+-------+
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
+-------+-------+-------+-------+-------+-------+-------+-------+-------+
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
+-------+-------+-------+-------+-------+-------+-------+-------+-------+
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
|       |       |       |       |       |       |       |       |       |
+-------+-------+-------+-------+-------+-------+-------+-------+-------+

Hexagonal Lattice

   +     +     +     +     +     +     +     +     +-----+-----+-----+---
                                                  /     /     /     /   
                                                 /     /     /     /   
+     +     +     +     +     +     +     +     +-----+-----+-----+-----+
                                                 \   / \   / \   / \   /
                                                  \ /   \ /   \ /   \ /
   +     +     +     +     +     +     +     +     +     +     +     +
                                                  / \   / \   / \   / \ 
                                                 /   \ /   \ /   \ /   \
+     +     +     +     +     +     +     +     +     +     +     +     +
                                                 \   / \   / \   / \   /
                                                  \ /   \ /   \ /   \ / 
   +     +     +     +     +     +     +     +     +-----+-----+-----+---
                                                    \     \     \     \ 
                                                     \     \     \     \  
+     +     +     +     +     +     +     +     +     +-----+-----+-----+

Note that there are three equivalent ways to orient the unit cells.

The 17 Plane Space Groups

Parallelogram Lattices

1. p1

  +     +     +     +     +     +     +     +     +     +     +     +     
                                      
   p     p     p     p     p     p     p     p     p     p     p     p
+     +     +     +     +     +     +     +     +     +     +     +     
                                       
 p     p     p     p     p     p     p     p     p     p     p     p
    +     +     +     +     +     +     +     +     +     +     +       

     p     p     p     p     p     p     p     p     p     p     p
  +     +     +     +     +     +     +     +     +     +     +     +

2. p2

  d           d           d           d           d           d     
    +     +     +     +     +     +     +     +     +     +     +
      p           p           p           p           p           p       
  +     +     +     +     +     +     +     +     +     +     +     +
          d           d           d           d           d           d     
      +     +     +     +     +     +     +     +     +     +     +
  p           p           p           p           p           p             
    +     +     +     +     +     +     +     +     +     +     +     +

There are 2-fold axes at the + locations.

Rectangular Lattices

3. pm

    p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
    b     b     b     b     b     b     b     b     b     b     b
---------------------------------------------------------------------
    p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
    b     b     b     b     b     b     b     b     b     b     b
---------------------------------------------------------------------
    p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
    b     b     b     b     b     b     b     b     b     b     b
---------------------------------------------------------------------

Parallel mirror planes

4. pg

    p     p     p     p     p     p     p     p     p     p     p
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
       b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
    p     p     p     p     p     p     p     p     p     p     p
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
       b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
    p     p     p     p     p     p     p     p     p     p     p
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
       b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

Parallel glide planes

5. pmm

+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+
|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|
+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+
|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|
+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+-----+

Perpendicular mirror planes

6. pmg

+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |    d|b    |
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|p    |    q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+

Perpendicular mirror and glide planes

7. pgg

+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |p   d|     |
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+
|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|     |b   q|
+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+- - -+

Perpendicular glide planes. (p,b) and (d,q) are related by horizontal glides, (p,q) and (b,d) by vertical glides. Note that the intersections of the glide planes and the centers of the boxes outlined by the glide planes are also two-fold symmetry axes.

Centered Lattices

8. cm

    p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
    b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
 p     p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
 b     b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
    p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
    b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
 p     p     p     p     p     p     p     p     p     p     p     p
---------------------------------------------------------------------
 b     b     b     b     b     b     b     b     b     b     b     b
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

Parallel mirror and glide planes. The pattern of stars on a 50-star flag has this symmetry.

9. cmm

+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+
|p :  |    q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|
+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+
|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|
+ -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- +
|  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |
+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+
|  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |
+ -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- +
|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|
+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+
|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|b :  |  : d|
+ -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- + -:- +
|  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |  : q|p :  |
+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+--:--+

Alternating mirror and glide planes in both directions. All intersections are also two-fold symmetry axes. Bricks in a wall have this symmetry.

Square Lattices

Trigonal Lattices

Hexagonal Lattices

Symmetry Elements of the Two-Dimensional Space Groups

Return to Symmetry Index
Return to Crustal Materials Index
Return to Professor Dutch's Home page

Created 17 Sep 1997, Last Update 22 Sep 1999

Not an official UW Green Bay site