We previously looked at what would happen if we had a residual (uncorrected) Cbar12 of < 1% (typical for our actual corrections) and tried to fit Cbar22 to find BPM tilts. The results were not promising (see here). Are we able to improve this quality-of-fit by utilizing all three measureable elements of the Cbar matrix?
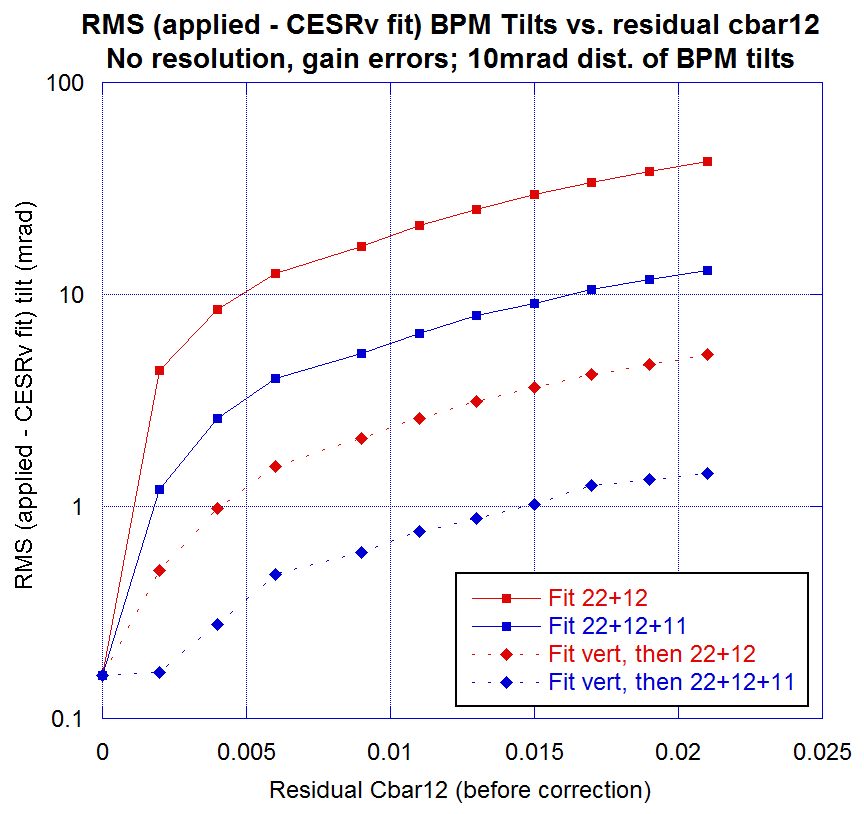
Using the now-standard setup (6x6 tracking, damp 40k turns, sample 16,384 turns) without resolution or gain errors, vary sk_q02w and record the resulting RMS Cbar12. For each set of data, fit using just Cbar12+22 and then using Cbar12+22+11 (restarting CESRv in-between fits for a fair assessment).
A vertical offset in a sextupole produces a skew-quad-like field. Therefore, it's theoretically possible to correct Cbar12 using vertical correctors.
Simulate: 6x6 tracking, damp 40k turns, sample 16,384 turns; vary sk_q02w as above; BPM tilt distribution of 10mrad. Fit Cbar12 with vertical steerings, then fit Cbar12 + 22 with BPM tilts.
Plot both sets of fits on the same graph:
By fitting against vertical steerings, then using all Cbar data, the typical residual Cbar12 of ~0.009 now yields a BPM tilt fit with a standard deviation of ~0.6mrad. If we can't use Cbar11 data, we are still able to fit within 2.1mrad.
Recall previous simulations of vertical dispersion measurements, on 2009.08.11. There, we looked at the effects of gain, tilt, and resolution errors on eta_y. In particular we were interested in what combinations of tilt and gain errors at 10um resolution would create an apparent 1cm eta_y (RMS) when actual eta_y = 0. (See the plot here). For an RMS agreement of 2.1mrad for BPM tilts, this means we must fit the gains to within ~0.66%.
These results are more or less independent of resolution for magnitudes we are interested in (< 35um). Recall this is because the Cbars themselves are insensitive to resolution errors due to the large amplitude of the measurement (~+/-6mm horiz, +/-4mm vert).