Physics 3316: Basics of Quantum Mechanics
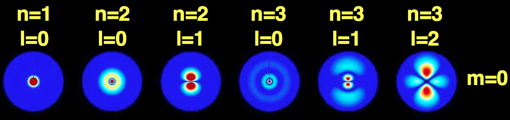
Probability density plots for some hydrogen wave functions (red=high). Imagine each plot to be rotated about the vertical z-axis.
This course is an introduction to quantum mechanics for undergraduate students. Topics include breakdown of classical concepts in microphysics; light quanta and matter waves; Schroedinger equation and solutions for square well, harmonic oscillator, and the hydrogen atom; wave packets, scattering and tunneling effects, angular momentum, spin, and magnetic moments.
Syllabus
- The experimental basis of quantum mechanics
- Introduction to wave mechanics
- Formalism
- Free particle, uncertainty principle, particle scattering
- Central potentials, Hydrogen atom
Suggested Literature
- An Introduction to Quantum Physics by French and Talyor
- Introduction to Quantum Mechanics, by D.J. Griffiths
- Quantum Physics of Molecules, Solids, Nuclei, and Particles, by R. Eisberg, and R. Resnick
- Quantum Mechanics, Concepts and Applications, by N. Zettili
- Night Thoughts of a Classical Physicist by R. McCormmach (fiction).
Lecture Notes
I do allow my lectures notes, syllabi, and other course materials to be freely shared and distributed under a Creative Commons Attribution-NonCommercial-ShareAlike license; please see below for details.
- Lecture 1: Introduction; double slit experiment
- Lecture 2: Photoelectric effect
- Lecture 3: Compton Effect
- Lecture 4: Blackbody radiation
- Lecture 5: Blackbody radiation, spectral lines
- Lecture 6: Bohr atom
- Lecture 7: de Broglie's particle waves
- Lecture 8: Particle waves, superposition and group velocity
- Lecture 9: Evidence for de Broglie waves; the "old quantum theory"
- Lecture 10: Approach to Schroedinger's Equation
- Lecture 11: Schroedinger's equation, wave function and probability
- Lecture 12: Expectation values e
- Lecture 13: Stationary states
- Lecture 14: Infinite square well
- Lecture 15: Finite square well, part I
- Lecture 16: Boundary conditions
- Lecture 17: Sketching stationary state wave functions
- Lecture 18: Finite well, part II
- Lecture 19: SHO potential
- Lecture 20: Numerical Solution of Schroedinger's equation
- Lecture 21: Formalism I
- Lecture 22: Formalism II
- Lecture 23: Formalism III
- Lecture 24: Dirac notation, 2-state systems
- Lecture 25: Photon polarization states I
- Lecture 26: Photon polarization states II
- Lecture 27: Momentum space wave function
- Lecture 28: Expectation values in momentum space
- Lecture 29: Free particle at t=0; uncertainty principle
- Lecture 30: Heisenberg's uncertainty principle
- Lecture 31: Free particle dynamics
- Lecture 32: Energy-time uncertainty, particle scattering
- Lecture 33: Step-up potential, probability current
- Lecture 34: Probability currents, scattering
- Lecture 35: Tunneling, QM in 3D
- Lecture 36: Separable potentials, central potentials
- Lecture 37: Angular momentum in QM
- Lecture 38: Angular momentum, spherical harmonics
- Lecture 39: Spherical harmonics, spin
- Lecture 40: Hydrogen atom
Creative Commons License
Attribution-NonCommercial-ShareAlike 3.0 United States
You are free:
- to Share: to copy, distribute, display my lecture notes and course materials
- to Remix: to make derivative works
Under the following conditions:
- Attribution: You must attribute the work in the manner specified by the author or licensor (but not in any way that suggests that they endorse you or your use of the work).
- Noncommercial: You may not use this work for commercial purposes Share Alike. If you alter, transform, or build upon this work, you may distribute the resulting work only under the same or similar license to this one.
For any reuse or distribution, you must make clear to others the license terms of this work. The best way to do this is with a link to this web page. Any of the above conditions can be waived if you get permission from the copyright holder. Apart from the remix rights granted under this license, nothing in this license impairs or restricts the author's moral rights.
Your fair use and other rights are in no way affected by the above. This is a human-readable summary of the Legal Code (the full license ).

Using an electronic ink/ interactive pen display in lecture.
